…On the Pythagorean Tradition
The outer form, or design, of a violin, has traditionally been seen as a reflection of its inner purpose — music. The elegant proportions, lines, and curves of a classic violin strike us with an immediate sense of easy grace and timeless perfection. We feel a harmony of design that we take in with our eyes as well as our ears, and which hints to us of a music that is deeper and more fundamental than that which our separate senses can discern.
The aesthetic traditions from which the violin’s design evolved have roots that can be traced back to some of the earliest expressions of our civilization. In ancient Greece, the concept of harmony was given a simultaneously musical and mathematical treatment by Pythagoras and his followers in the sixth century B.C.
Ancient Greek legend tells us that Pythagoras was one day walking by a forge where the smith was pounding away at the anvil with set of hammers. Pythagoras paused to listen to the ringing of the hammers and the melody that was being played between them. His curiosity aroused, he enquired of the smith as to the nature these hammers that they made such a harmonious concordance. He discovered that the weights of the hammers were in the ratio of 6,8,9 and 12 pounds, and together they rang out with the tones of a fundemental with its fourth, fifth, and octave. (That is, a base tone along with those four, five and eight notes above on the musical scale — do, fa, sol, do) At this, Pythagoras was struck with a revalation that it was mathematical harmony as exemplified in music that was the common power uniting the heavens and the earth.
This Pythagorean legacy — the idea of the centrality of music and its mathematical expression to our understanding of the order of nature — became a key concept in music and art, science and philosophy for many centuries afterwards. Indeed, its influence is felt even in modern times as an archetype of the attempt to find a rational order behind the chaos of immediate experience.
To briefly trace this tradition in order to understand some of its influence on the art of lutherie, let us look at a couple of concepts that were first given expression by the ancient Greeks: ‘harmony’ and ‘proportion’ — words that have for millennia been used to describe the defining characteristics of the idea of the beautiful in classical aesthetics.
The word harmony originally had the meaning of joining or fitting together, much as a carpenter joins two pieces of wood to make one. It is interesting to note also that our word ‘art’ can be traced back to the same root meaning. For the Greeks, beauty, harmony, and proportion were very closely related ideas. A beautiful object exhibited an inner harmony or wholeness that arose from its parts being joined together in proper proportion. Analogously, our concept of rationality is similarly connected to the mathematical idea of ratio.
The Greek philosophers were known for their clear and rational thought, and proportion to them had a very specific mathematical connotation, and how they thought about it in connection to the abstract idea of harmony is especially revealed in the doctrine of what they called the ‘means’.
In early Pythagorean thought, a mathematical ‘mean’ or ‘mean proportional’ concerned the division of a quantity, or line segment, into sections that are in a clearly defined and simple ratio. Between two extremes, or outer terms of a given ratio, a third, or mean, term is placed; and by its relationship to the two extremes, an organized mathematical progression is created. A harmony or wholeness is thereby brought into the progression by virtue of these mutual relationships; the extremes are harmonized by their relationship to the mean or middle term and the whole and its parts are brought into a well proportioned harmony. For example, let us take two terms of a ratio, one of which is twice the other, in this case 6 and 12. A mean term placed between these two extremes divides the ratio into two proportions fitting together with a specific inner harmony. In the case of what was called the arithmetic mean, the mean term divides the extremes so that each term exceeds the previous by the same amount – such as in the progression 6:9:12, where 9 exceeds 6 by 3 and 12 exceeds 9 by 3. In other words, the terms increase in a simple arithmetic progression by equal increments. In this case, the mean divides the original double ratio of 6:12 into the ratios 6:9 and 9:12. Reduced to lowest terms, we can say that 1/2 divided by the arithmetic mean, yields 2/3 and 3/4.
With what was known the harmonic mean, the mean term is distant from each of the extremes by the same fraction of that extreme. For example, between 6 and 12, the harmonic mean is 8. So in the progression 6:8:12, the mean term 8 is greater than 6 by 1/3 of 6 and is less than 12 by 1/3 of 12. Here we have the inverse of the arithmetic mean, and we arrive at ratios which reduce to 3/4 followed by 2/3. In this whole series then, (applying both the arithmetic and harmonic means) we have 6:8:9:12 — or, in equivalent fractional terms — 1/2, 2/3, 3/4, and1.
If you have followed this, you will see that these proportions are the same as those discovered by Pythagoras in the ringing hammers. The Pythagoreans further experimented with the one string monochord to analyze the intervals of their early musical scale by comparing the ratios of string lengths. Here again the same ratios, generated from the proportions of the arithmetic and harmonic means, were revealed in the tones of music. The rational abstract harmonies of pure mathematics were given life in the intuitive sense of musical consonance, and were empirically discovered in the weights of the smiths’ hammers and in the lengths of the vibrating strings. Music for them revealed the simple hidden harmony that lay behind all the diversity of nature. The interval of an octave, the most fundamental and intuitive of musical phenomena, had a ratio of 1 to 2,. and by dividing the octave by the arithmetic and geometric mean, the perfect consonances of the fifth and fourth arose. Later, it was found that when the arithmetic and harmonic means are applied to the interval of the fifth (ratio 2:3) rather than the octave (1:2), the imperfect consonances of the minor and major thirds (ratios 4:5 and 5:6) were obtained. The interval between the arithmetic and harmonic mean, in the case of the octave, is the whole tone; in the case of the fifth, it is semitone. These, then, were the roots of the intervals of our musical scale, in what is probably one of their earliest expressions, arrived at by dividing the octave by the harmonious ratios of the mean proportionals.
When we examine these ratios, we find a natural hierarchy, reflected in the harmony of the scale, that is based on the simple progression of numbers from unity to complexity. From the perfect consonances of the unison and its octave (expressed as 1:1 and 1:2) we pass to the ratios of 2:3, the musical interval of the fifth, which functions musically as the opposite pole of the harmonic spectrum in its role as the dominant degree of the scale, and is the next most consonant, and fundamental interval; and its inverse, the fourth, or subdominant, with a ratio of 3:4. For many centuries these were the only intervals that were considered to be consonant, and they became the foundation of musical theory and practice as well as the classical theory of aesthetic proportion in other arts as well. As the musical and theoretical vocabulary expanded around the time of the Renaissance, the thirds (with ratios of 4:5 and 5:6) and finally the sixths (3:5 and 5:8) were taken into the family of accepted consonant intervals. It can be seen from all this that the fundamentals of music are built from the simplest elements of mathematics, and that they fall into a natural hierarchy beginning in the perfect consonance of the unison and octave, and steadily falling off into dissonance as they unfold into complexity.
Now there is one more species of mean proportional that should also be mentioned, which is relevant to our topic, and that is what has come to be known as the ‘Golden Mean’ the proportions of which may also be familiar as found in golden rectangles, or golden spirals which are generated from this ratio. The golden mean has been the subject of much poetic speculation, to the point of mystical attributions. The golden mean, or golden section, divides a line segment such that the smaller part is in the same ratio to the larger part, as the larger part is to the whole. Its special significance seems to be that in carrying the proportion between the parts outward to the whole, we introduce a new dimension of self-reflection, of the echoing of the whole within the parts, and the intimations of infinity. Many natural phenomena, most notably the spiral of a conch shell, the pattern of seeds in a sunflower, or the arrangement of leaves on many plants, exhibit a self-replicating pattern of growth reflecting this ratio. The golden mean also marks the intersections of the arms of the five-pointed star, or pentagram, which was the special symbol of the early Pythagoreans; and its proportions have been used in the laying out of architectural facades dating back at least as far as the Greek Parthenon..
Solved for numerically, the golden mean yields a never ending, irrational number in the region of .618…… and as a musical interval has no practical application; but theorists of earlier and more poetic ages did not let that stop them from laying claim to its special qualities as a symbol of a higher, more perfect harmony, to be heard not with earthly ears, but with those of the spirit.
150 years after Pythagoras, Plato took up much of the Pythagorean doctrine. His eternal Ideas or Forms, of which earthly objects were mere shadows, found their perfection in the timeless truths of mathematics; and music was the bridge between the temporal world and that of the pure and timeless Ideas. In his book the ‘Timaeus’, Plato describes the architect of the world bringing order out of chaos by dividing up and laying out the heavens and the earth in proportion, according to the musical ratios. Music was an all pervading force of nature — a world harmony that guided the planets in their courses, the seasons on the earth, and the ordering of society and the human soul. Just as our modern conception of science relies on simple mathematical laws to describe the phenomena of nature, so in the Pythagorean view, the simple ratios of the musical intervals were the outward sign of a deeper harmony that in essence guided all natural relations.
From Plato and the Pythagoreans, the concept of a mathematically based musical harmony as the great ordering principle of the world was passed on to the scientists, philosophers and artists of succeeding ages. Ptolemy, whose astronomical world system held sway until the time of the Copernican revolution, described in his book the ‘Harmonics’ a cosmic harmony that ordered the movements of the stars and planets around the earth by the now classical musical ratios. But it was Augustine, and following him, Boethius, in the early years of the Christian era, whose books on music influenced the ideas and practice of artists and architects throughout the middle ages, formulating aesthetic ideals based on the expression of cosmic harmony that would lead the mind to contemplation of the divine order. The geometry of the simple musical consonances was taken to be the earthly image of a greater eternal harmony, and the perfection of these proportions was obvious not only to the ear, but to the eye as well. The well worn description of architecture as ‘frozen music’ is nowhere truer than in the most magnificent creations of the middle ages, the great Gothic cathedrals, such as the ones at Chartres and Rheims in France, where the musical ratios of the perfect consonances were quite explicitly incorporated into the proportions of the buildings. These cathedrals stood as models of the medieval universe, reflecting the inner harmony of the cosmos in the terms of music, and sharing the same mathematical vocabulary.
As the Renaissance began to flower in Italy, a newly reinvigorated examination of the early Greek writings infused the intellectual climate of the times with a keen interest in the Platonic and Pythagorean ideas on philosophy and aesthetics. At the beginning of the Renaissance, Phillippo Bruneleschi, the ‘father of Renaissance architecture’ became well versed in the musical geometry of the ancients, and quite clearly incorporated their proportions into his work. Leon Battista Alberti, who wrote treatises on both architecture and painting which were widely circulated amongst the artists of the time, went into detail describing the simple proportions of the intervals of the musical scale as the key to the harmonious composition of spaces in architecture and in painting.
One interesting painting of that time is Raphael’s ‘School of Athens’ which presents us with many of these ideas. In this painting, the central figures are Plato and Aristotle. Plato, the mystic and idealist, points upwards; Aristotle, the earthly realist is pointing downwards. On Plato’s side, in the foreground on the left is Pythagoras, contemplating a diagram outlining the relationships of his musical intervals; on the side of Aristotle, in the foreground on the right, geometric figures are being marked out on the ground with a compass. The composition of the painting as a whole can be seen to be symmetrically organized, from the proportions of the canvas to the placement of the elements within it, by the simple musical ratios.
In the same year as Raphael’s painting, a book was published in Venice by Fra Luca Pacioli, which was entitled ‘The Divine Proportion’ which extolled, in almost mystical terms, the mathematical and aesthetic beauties of the golden section, or golden mean, and its mysterious properties. It was illustrated with geometric drawings by Leonardo DaVinci, who was also known to proclaim music to be the sister of painting.
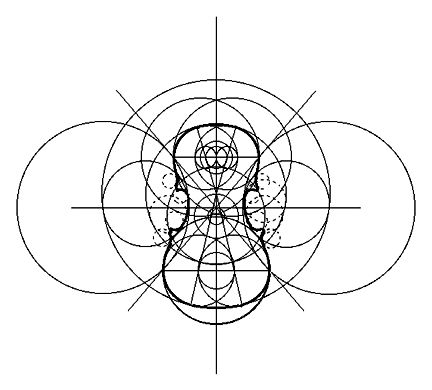
Such was the intellectual climate of renaissance northern Italy, at the beginning of the 16th century. It was here at this time that the violin first evolved from the earlier medieval viols. The idea of spatial design guided by the traditional musical ratios was not new, and there is evidence of its use by the makers of lutes and viols in the late middle ages and early renaissance as well. The luthiers and violin makers of the time participated in these ideas, and it would have only seemed natural that an object designed to create music in the worldly sense would incorporate the classical principles whereby it would reflect also the cosmic harmony that echoed throughout nature and society. The early violin makers, in working out their designs, would have had a background in the idea that the outward design of their instruments should naturally reflect the inner musical voice, which in turn was the reflection of Nature’s original harmony, and to which geometry was the key. And so with compass and straightedge, the renaissance designers laid out the flowing arcs and eloquent proportions of the violin, incorporating in various ways the classical mathematical ratios.
In our own day, while we have divorced our aesthetic sense from that grand vision of an all embracing harmony, the Pythagorean legacy stills lives on in our mathematical sciences. The acoustical foundations of music — the phenomena of periodic vibration, resonance, and the mathematical description of these, are concepts fundamental to our modern understanding of chemistry, physics, engineering, and astronomy. For the modern luthier, while the discipline of acoustical physics has supplanted the older ideal of musical proportion as a way of understanding our work, the Pythagorean ideal still has relevance. Some luthiers yet try to capture the soul or character of an instrument in number, such as in the equations expressing the simple relationships between mass, stiffness, and dimension that determine its resonant frequencies and tonal response.
But whether beauty can ever really be captured by our mathematical reasoning is doubtful, and perhaps the Pythagorean vision is best left as it always has been — a poetic ideal and an inspiring metaphor for the imagination.
References:
Lippman, Edward A: Musical Thought in Ancient Greece ; Columbia University Press, 1969
Crocker, Richard L: Pythagorean Mathematics and Music ; Journal of Aesthetics and Art Criticism , Winter 1963
Helm, Eugene: The Vibrating String of the Pythagoreans ; Scientific American , December 1967
Von Simson, Otto: The Gothic Cathedral ; Bollingen, 1962
Wittkower, Rudolf: Architectural Principles in the Age of Humanism ; St Martin’s Press, 1988
Bouleau, Charles: The Painter’s Secret Geometry ; Harcourt, Brace, 1963
Coates, Kevin: Geometry Proportion and the Art of Lutherie ; Oxford, 1985